Coordonnées cartésiennes vers polaires
\iint_E f(x,y) \;\mathrm dx\mathrm dy = \iint_F f(r\cos\theta,r\sin\theta)r \;\mathrm dr\mathrm d \theta


Exemple  :

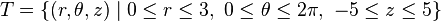
Passage d'un anneau de coordonnées cartésiennes à polaires

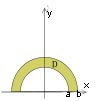
Considérons $\{(x,y) | 4 = x² + y² = 9, y = 0\}$, soit une demi-couronne de rayon extérieur 3 et de rayon intérieur 2 (voir dessin), limitée aux $y$ positifs ; on voit que $\theta$ varie de 0 à $\pi$ et $r$ de 2 à 3. Par conséquent, le domaine transformé en coordonnées polaires est le rectangle $\{(r,\theta) | 2 < r < 3, 0 < \theta < p\}.

Soit $f(x,y) = x$ à intégrer sur ce domaine f(x,y) = x \longrightarrow f(r,\theta) = r\cos \theta;

puis appliquons la formule pour l'intégration :

\iint_D x \;\mathrm dx\mathrm dy = \iint_T r\cos\theta\,r\;\mathrm dr\mathrm d \theta = \iint_T r^2\cos\theta\;\mathrm dr\mathrm d \theta.

Enfin :

\int_0^\pi\mathrm d\theta\int_2^3 r^2\cos\theta\;\mathrm dr = \int_0^\pi\cos\theta\;\mathrm d\theta \left[ \frac{r^3}{3}\right]_2^3 = [\sin\theta]_0^\pi \left(9 - \frac{8}{3}\right) = 0.

Coordonnées cartésiennes vers cylindriques
\iiint_D f(x,y,z) \;\mathrm{d}x \mathrm{d}y \mathrm{d}z = \iiint_T f(r \cos \theta, r\sin \theta, z) r \;\mathrm{d}r \mathrm{d}\theta \mathrm{d}z


Il est conseillé d'utiliser cette méthode dans les cas de domaines cylindriques, coniques, ou tout du moins de régions pour lesquelles il est commode tant de délimiter l'intervalle des z que de transformer la base circulaire et la fonction.

Exemple  :

Soit f(x,y,z) = x2 + y2 + z à intégrer sur le cylindre {{math|D = { (x,y,z) | x² + y² = 9, -5 = z = 5 }}}. La transformation de D en coordonnées cylindriques est la suivante :

T = \{ (r,\theta,z)\mid 0 \le r \le 3, \ 0 \le \theta \le 2 \pi, \ -5 \le z \le 5 \}

tandis que la fonction devient

$f(r,\theta,z) = r^2 + z$

Appliquons la formule :

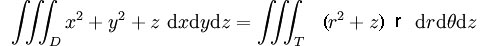\iiint_D x^2 + y^2 +z \ \mathrm{d}x \mathrm{d}y \mathrm{d}z = \iiint_T r^2 + z \ \mathrm{d}r \mathrm{d}\theta \mathrm{d}z;

En développant :

\int_{-5}^5 \mathrm{d}z \int_0^{2 \pi} \mathrm{d} \theta\int_0^3 r^3 + r z\; \mathrm{d}r = 2 \pi \int_{-5}^5 \left[ \frac{r^4}{4} + \frac{r^2 z}{2} \right]_0^3 \mathrm{d}z = 2 \pi \int_{-5}^5 \left( \frac{81}{4} + \frac{9}{2} z\right) \mathrm{d}z = \ldots = 2 \pi \left(\frac{405}{2} + 225\right).

Coordonnées cartésiennes vers sphériques (on posera: $\rho = r$)
\iiint_D f(x,y,z) \;\mathrm{d}x \mathrm{d}y \mathrm{d}z = \iiint_T f(\rho \sin \theta \cos \phi, \rho \sin \theta \sin \phi, \rho \cos \theta)\,\rho^2 \sin \theta \;\mathrm{d} \rho \mathrm{d} \theta \mathrm{d} \phi


Il est conseillé d'utiliser cette méthode dans le cas de domaines sphériques et de fonctions facilement simplifiables à l'aide des identités trigonométriques étendues à R³ (voir l'exemple suivant) ; dans les autres cas il est souvent conseillé de recourir au passage en coordonnées cylindriques, comme nous le verrons ci-après.

Exemple  :

Soit $f(x,y,z) = x^2 + y^2 + z^2$ à intégrer sur le domaine $\{(x,y,z) | x² + y² + z² = 16\}$ (sphère de rayon 4 centrée à l'origine). La transformation de la fonction est très simple:

f(r \sin \theta \cos \phi, r \sin \theta \sin \phi, r \cos \theta) = r^2~,

tandis le domaine reformulé en coordonnées sphériques est le suivant:

T = \{(\rho,\theta,\phi)\mid 0 \le \rho \le 4, 0 \le \phi \le 2 \pi, 0 \le \theta \le \pi\}.

Appliquons donc la formule pour l'intégration :

\iiint_D x^2 + y^2 +z^2 \;\mathrm{d}x \mathrm{d}y \mathrm{d}z = \iiint_T \rho^2 \,\rho^2\sin\theta \;\mathrm{d}\rho\mathrm{d}\theta\mathrm{d}\phi;

développons :

\iiint_T \rho^4\sin\theta \;\mathrm{d}\rho\mathrm{d}\theta\mathrm{d}\phi = \int_0^\pi \sin\theta\mathrm{d}\theta \int_0^4 \rho^4\mathrm{d}\rho \int_0^{2\pi} \mathrm{d} \phi = 2 \pi \int_0^\pi \sin \theta\left[ \frac{\rho^5}{5}\right]_0^4 \;\mathrm{d} \theta= 2 \pi \left[ \frac{\rho^5}{5} \right]_0^4 \;[- \cos \theta ]_0^\pi = 4 \pi \cdot \frac{1024}{5} = \frac{4096 \pi}{5}.
© Copyright - Source : article Intégrales multiples sur Wikipédia - Cet article est sous licence GFDL